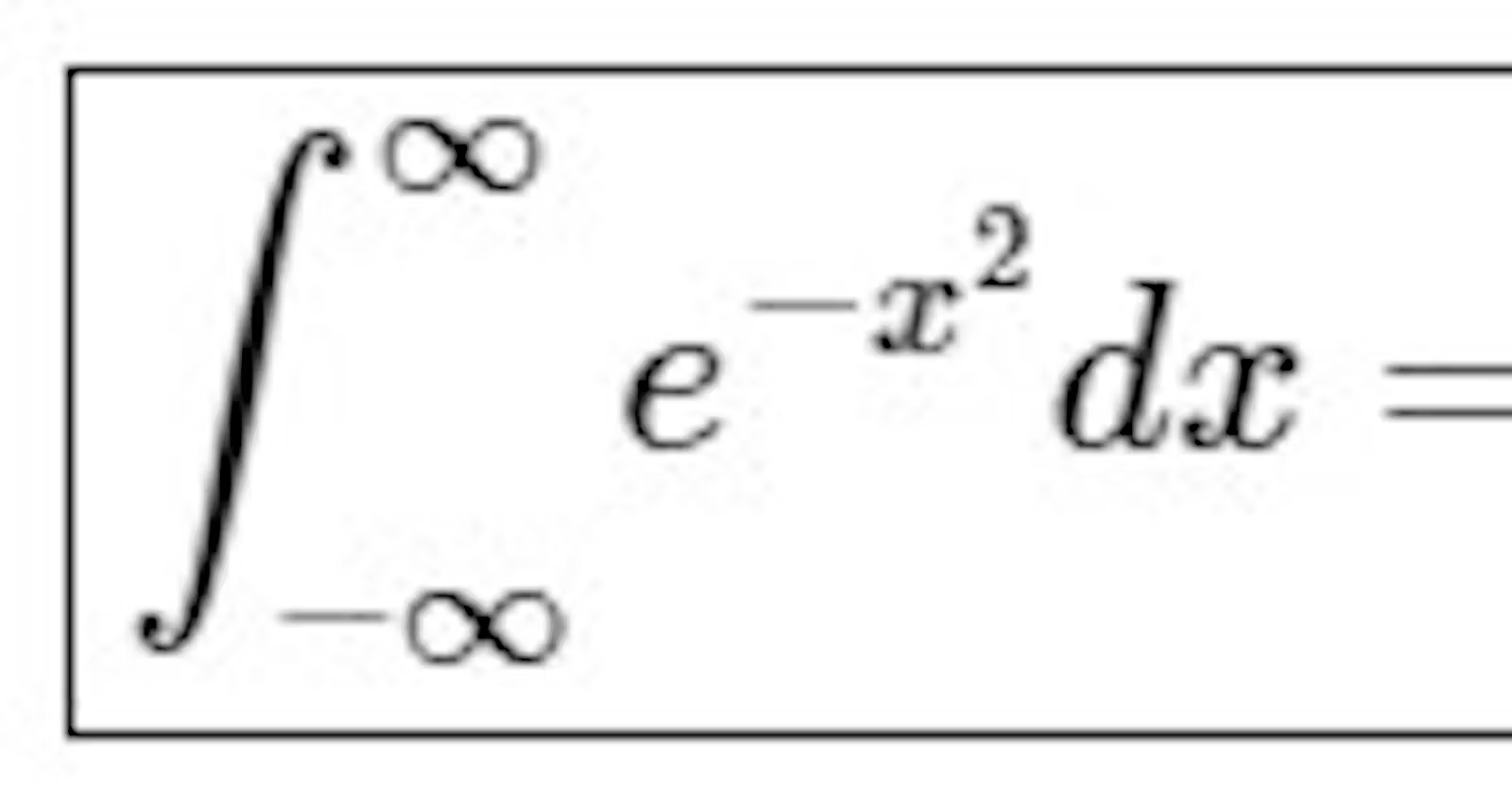The definite integral of $e^{-x^2}$ over the entire real line does have a beautiful result involving $\pi$:

$$\boxed{{\displaystyle {\int }_{-\mathrm{\infty }}^{\mathrm{\infty }}{e}^{-x^2}dx=\sqrt{\pi }}}$$

Consider the square of the integral: Let ${\displaystyle I={\int }_{-\mathrm{\infty }}^{\mathrm{\infty }}{e}^{-x^2}dx}$, then${\displaystyle I^2={\int }_{-\mathrm{\infty }}^{\mathrm{\infty }}{e}^{-x^2}dx{\int }_{-\mathrm{\infty }}^{\mathrm{\infty }}{e}^{-x^2}dx}$

Use Fubini’s theorem to convert to a double integral: ${\displaystyle I^2={\int }_{-\mathrm{\infty }}^{\mathrm{\infty }}{e}^{-x^2}dx{\int }_{-\mathrm{\infty }}^{\mathrm{\infty }}{e}^{-y^2}dy}$

Simplify and convert to polar coordinates: ${\displaystyle I^2={\int }_{-\mathrm{\infty }}^{\mathrm{\infty }}{\int }_{-\mathrm{\infty }}^{\mathrm{\infty }}{e}^{-(x^2+y^2)}dxdy}$

Now, convert to polar coordinates:

$$\begin{array}{rl}& x=r\cos \theta \\ & y=r\sin \theta \\ & dx.dy=r.dr.d\theta \end{array}$$

So $I^2={\displaystyle {\int }_0^{2\pi }{\int }_0^{\mathrm{\infty }}{e}^{-r^2}rdrd\theta }$

Evaluate the double integral: First, evaluate the inner integral with respect to $r$. We can use a simple substitution: let $u=r^2,du=2rdr$

$${\displaystyle {\int }_0^{\mathrm{\infty }}{e}^{-r^2}rdr=\frac{1}{2}{\int }_0^{\mathrm{\infty }}{e}^{-u}du=\frac{1}{2}{[-e^{-u}]}_0^{\mathrm{\infty }}=\frac{1}{2}}$$

Now, substitute this back into the double integral:

$$I^2={\int }_0^{2\pi }\frac{1}{2}d\theta =\frac{1}{2}[\theta {]}_0^{2\pi }=\pi$$

Take the square root $I^2=\pi ⟹I=\sqrt{\pi }$. So we have proven that:

$$\boxed{{\displaystyle {\int }_{-\mathrm{\infty }}^{\mathrm{\infty }}{e}^{-x^2}dx=\sqrt{\pi }}}$$